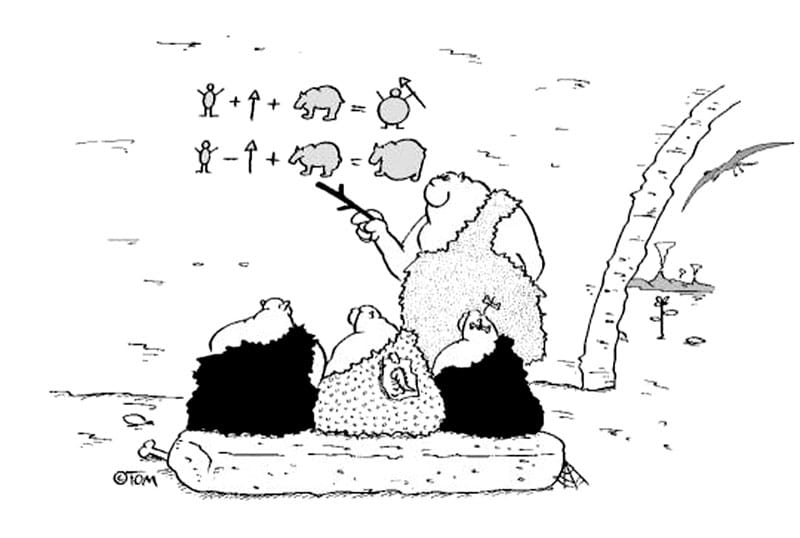
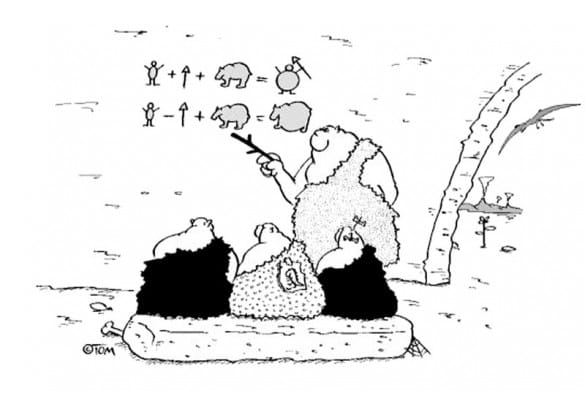
I like to tell people I am numerically dyslexic. When I look at a page of numbers, for example a spreadsheet, all I can see is a load of numbers lying around saying nothing to me. My eye simply falls to the bottom right-hand corner, then, if it likes the number it finds there, it slowly works its way back up the sheet to see where the number came from. As it does so, the numbers seem to jump to attention and start talking. Then I don’t understand what they are saying so I ask my accountant what’s going on. Then I look confused and I ask if we’re making money or losing money. I think this is caused by lack of interest more than anything else. I’m fairly sure I have the mental capacity to be better at maths (or “math” for the Americans) than I am, but I am just not interested. I prefer words.
But I am fascinated by what can happen with numbers. There are disputes, laws and absolutes that seem impossible to the average idiot (like me). For example, what would you say if I told you that .9999 (recurring) is equal to one? Most people would say I’m proving how mathematically challenged I am. Let me try to explain the argument; what is “one third” expressed as a decimal? It’s .3333 (recurring), right? And three “thirds” added together make a whole “one”. Multiply .3333 (recurring) by three in the same way and you get .9999 (recurring). So .9999 (recurring) is equal to one. If I cut a cake into three equal pieces and gave three people one piece each they’d each have 33.3333 (recurring) percent of the cake. If all three people gave their piece of the cake back to me, I would again have 100% of the cake. Not 99.9999 (recurring) percent. What’s really fascinating is that great mathematicians from all over the world debate this issue over and over again. How can there be any uncertainty in math(s)? At the root of this debate is the concept of infinity. That’ll keep you glued to your computer screen for a few more hours (and for once it won’t matter if somebody catches you).
How about this: Imagine you are on a game show and you are told that one of three doors in front you has a million dollar prize behind it. The other two doors have nothing behind them. You must choose a door. After you have chosen your door (but not yet opened it), the host will open another one of the doors, show you there is nothing behind it and ask you if you wish to stay with your first choice of door or switch to the other closed door. Either the door you chose or the last one of the three definitely has a million dollars behind it. What would you do? Stay with your first choice or switch? If you’re like me you’ll think it’s a 50 / 50 choice, assume there’s a reason why they want you to switch and stick to your first choice. But we’d probably be wrong. The fact is, if you stay with your first choice you have roughly a one in three (33.3333 percent) chance of being right, but if you switch you have a roughly two in three (66.6666 percent) chance of being right. So, you are twice as likely to win the million dollars if you switch. Google “Monty Hall problem” for an explanation. Amazing and completely counterintuitive.
If you knew and understand all of the above already then you are a real smarty pants and you probably got beaten up a lot at school. There’s always .9999.